等分除と包含除，それと不名数―Klapper (1916)より

年始に2chからアクセスがありました．見てみると，http://wc2014.2ch.net/test/read.cgi/math/1448088399/の543と550で，同一IDの人がURLを書いてくださっています．また555には，Vergnaud (1983)の私訳が転載されていました．
少し手を広げて，情報散策をしていると，次の記述を目にしました．

「名数，不名数」についてのメモ（その1） | メタメタの日

わり算の意味をこのように2通りに区別することは，高木だけでなく，この時代の通則であり，教育学者・教師の間では，
　（1）名数÷名数＝不名数（24円÷6円＝4）のタイプは，「包含除」，
　（2）名数÷不名数＝名数（24円÷6＝4円）のタイプは，「等分除」
と呼ばれるようになり，「包含除，等分除」の用語は，現在の算数教育界にも引き継がれている。しかし，この用語は日本独特らしい。銀林浩さんによれば，「包含除，等分除」に対応する用語は，ドイツ語にはあるが，英語やフランス語にはないらしい。
(略)

日本独特ではないことは，英語で（日本人以外によって）書かれた論文やWebの情報を見れば容易に確認できますが，上記の中でまず目にとまったのは「フランス語」でして，Vergnaud (1983)を読み直しました．もちろんこれは英語ですが，そこから，フランス語には「包含除」「等分除」に対応する語がないかもしれないなと推測ができます．
それぞれに対応する概念は，明示されています．等分除を表すのがfirst-type division，包含除はsecond-type divisionです．例題を書き出しておくと，前者は"Example 3. Connie wants to share her sweets with Jane and Susan. Her mother have her 12 sweets. How many sweets will each receiver?"，後者は"Example 5. Peter has $15 to spend and he would like to buy miniature cars. They cost $3 each. How many cars can he buy?"です．
ただ，first-type divisionとsecond-type divisionが，multiple proportion（複比例）の話でも出てきて，それらは包含除・等分除に割り当てられません．これも，例題を書いておきますと，順に"Example 14. A farmar tries to calculate the averageproduction of milk of his cows during the 180 best days of the year. With 17 cows, he has produced 70,340 liters of milk during that period. What is the average production of milk per cow per day?"，"Example 15. A scout camp has just received 500 kg of cereal. The allowed distribution of cerial is 0.6 kg per person per week. There are 236 persons in the camp. How long will the cereal last?"です．
いずれも，最も大きい数を他の2数で割れば，答えが出てきますが，単位の扱いが異なっています．実際，Example 14では70340[kg]÷180[日]÷17[頭]≒23[kg/頭・日]と表せるのに対し，Example 15だと500[kg]÷0.6[kg/人・週]÷236[人]≒3.5[週]となります．
ここで「23[kg/頭・日]」や「0.6[kg/人・週]」は，「複内包量」です（もちろん当該文献には出てきませんが）．この用語を使って違いを説明するなら，前者の例題は複内包量を求めるもので，等分除を複比例の場面に適用したと見ることができるのに対し，後者の例題は，除数の1つに複内包量が入っており，異なる演算となっています*1．
英語論文での「包含除，等分除」の使われ方については，次のものが分かりやすいと思います．ログインすれば無料で全文が読めます．*2

Fischbein, E., Deri, M., Nello, M. S. and Marino, M. S. (1985). The Role of Implicit Models in Solving Verbal Problems in Multiplication and Division. Journal for Research in Mathematics Education, Vol.16, No.1, pp.3-17. http://www.jstor.org/stable/748969

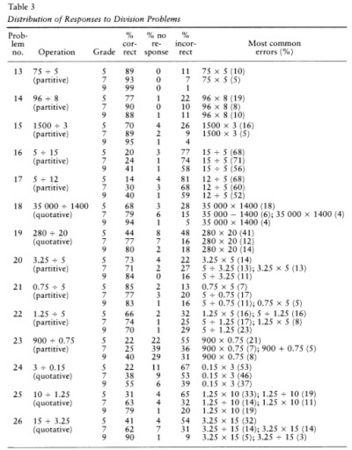
"(partitive)"と"(quotative)"が，それぞれ等分除，包含除です．本文でPartitive divisionとQuotative divisionの定義*3が書かれ，調査に用いた問題も別途，表になっています．例えばProblem no.16の問題は"15 friends gether bought 5 kg of cookies. How much dideach one get?"で，17は，「15」を「12」に変えただけです．2種類の問題セットを用意して解かせています．16と17の問題について，他よりも正解率("% Correct")が低くなっているのは，わられる数とわる数を逆（大÷小）にしてしまいやすいからで，日本でも，全国学力テストに類題がありました*4．
もっと最近の海外情報からだと，Common Coreも見ておきたいところです．CCSS.Math.Content.3.OA.A.2では2種類のわり算の場面が例示され，順に等分除と包含除です．またアレイ図の改訂で（年明けに）リンクしたTable 2では，各場面を，乗法・等分除・包含除の3つの列で構成しています．

今からちょうど100年前の，米国の書籍にも，等分除・包含除が載っていました．

Klapper. P. (1916). The teaching of arithmetic. D. Appleton and company. https://archive.org/details/teachingarithme00klapgoog

wikipedia:除法，wikipedia:en:Division_(mathematics)，wikipedia:en:Quotition_and_partitionを見ていくと，Referencesに書かれています．あとは検索で，上記ページを知りました．著作権は切れており，PDF，EPub，Kindleなどのファイルがダウンロードできます．
本文p.202（PDFのページでは217）に，Quotition and Partitionから始まる項目があります．

Quotition and Partition.---Division may take one of two forms. Let us assume that a given whole must be divided into a number of groups; it may become necessary to find the number of units in each group. If 16 cents is to be divided among a number of children, each receiving 2 cents, then it is necessary to find the number of children who will be given 2 cents each, but if 16 cents is to be distributed among 8 children, then it is necessary to find the number of cents that will be given to each child. These two forms of division are known as quotition and partition respectively. In quotition the number of equal groups is sought; the numbers in the dividend and in the divisor refer to the same commodity; the numbers in the dividend and in the quotient refer to different commodities. In partition, the number of units in each equal group is sought; the number in the dividend and in the quotient refer to the same commodity; the number in the divisor is abstract. Partition is a form of division more closely related to finding fractional parts of a number, e.g., " $\frac15$ of $15"; quotition is a form of division more closely related to problems which find what fractional part one quantity is another, e.g., "$3 is what part of $15" While there two forms of division must be understood by the teacher and should be given to the class in the variety of examples offered for solution, there terms should never be taught to the children.

訳してみます（適宜改行しました）．

等分除と包含除

　わり算には2種類があると考えられる．与えられた全体（の数や量）を，多数のグループに分ける状況を仮定しよう．各グループの1つ分の数を求める必要があるかもしれない．16セントを多くの子どもたちに分ける場合，1人につき2セントずつ受け取るとすれば，2セントを受け取る子どもの人数を求めることになる．しかし16セントを8人の子どもたちに（等しく）配るなら，それぞれの子どもに与えるのが何セントかを求める必要がある．
これら2種類のわり算は，それぞれ包含除，等分除として知られている．
　包含除では，（同じ数量になる）グループの数を求める．被除数と除数は同じ種類の量となる．被除数と商は異なる種類の量となる．
　等分除では，（同じ数量になる）グループの，1つ分の数量を求める．被除数と商は同じ種類の量となる．除数は抽象数となる．
　等分除は，「15ドルの $\frac15$ 」といった，ある数（量）の決まった分数部分（割合）を求めることと密接に関係する．包含除は，「3ドルは15ドルの何倍」のように，ある数（量）が他方の何倍かを求めることと密接に関係する．
　これら2種類のわり算について，教師は理解して授業においては様々な例とともに求め方を教えるべきである．ただしそれらの用語を子どもたちに教えるべきではない．

内容は，上記や現在の「等分除」「包含除」と同じと言っていいでしょう．そして"the number in the divisor is abstract."におけるabstractが，不名数*5のことだと思えば，日本の算術と，話がつながってきます．
Klapperの本で，abstractの定義を見つけられていませんが，乗法の交換法則を説明しているp.185（PDFのページでは200）にも，"The law of commutation in arithmetic is summed up in the equation a×b＝b×a when both a and b are abstract."と，abstractが出現します*6．Anghileri & Johnson (1988)で見てきた"Note that this is a property of numbers. While it is true that 3×4 is equal to 4×3, 3×4 may not be the same as 4×3 in a real-life situation."と，重なってきます．

わさっきhb

大学（教育研究）とか，親馬鹿とか，和歌山とか，とか，とか．

等分除と包含除，それと不名数―Klapper (1916)より

等分除と包含除

関連